After receiving the mid-term exam paper, J.L., a student in my math class, cried to me, “I’ve tried my best to learn geometric proofs, but I just don’t know when and where to add auxiliary lines. I’m not as smart as D.G. (D.G. is the best performer in the class). Mathematics frustrates me”. J.L. is one of the many students who have similar struggling experiences when learning mathematics, especially in the transition from primary school to secondary school. Some of the students overcome the challenges and move on, but others hold the stereotype that math only belongs to those smart minds and stop putting effort into the subject. Those who believe that math is based on one’s innate ability and that efforts don’t make much difference are said to have a fixed mindset, which negatively affects their study and life (Dweck). On the other hand, a growing mindset enables students to take challenges and work hard consistently to acquire specific abilities. Therefore, it is vitally important to prepare students with a growing mindset, which is almost common sense nowadays. Nevertheless, the theory does little to help ease the “math hurts”, particularly in high-demanding and competitive test-oriented learning environments like East Asia. To cultivate a growing mindset, students first need to prepare for the challenges with positive mental attitudes towards practice, mistakes, and self-growth.

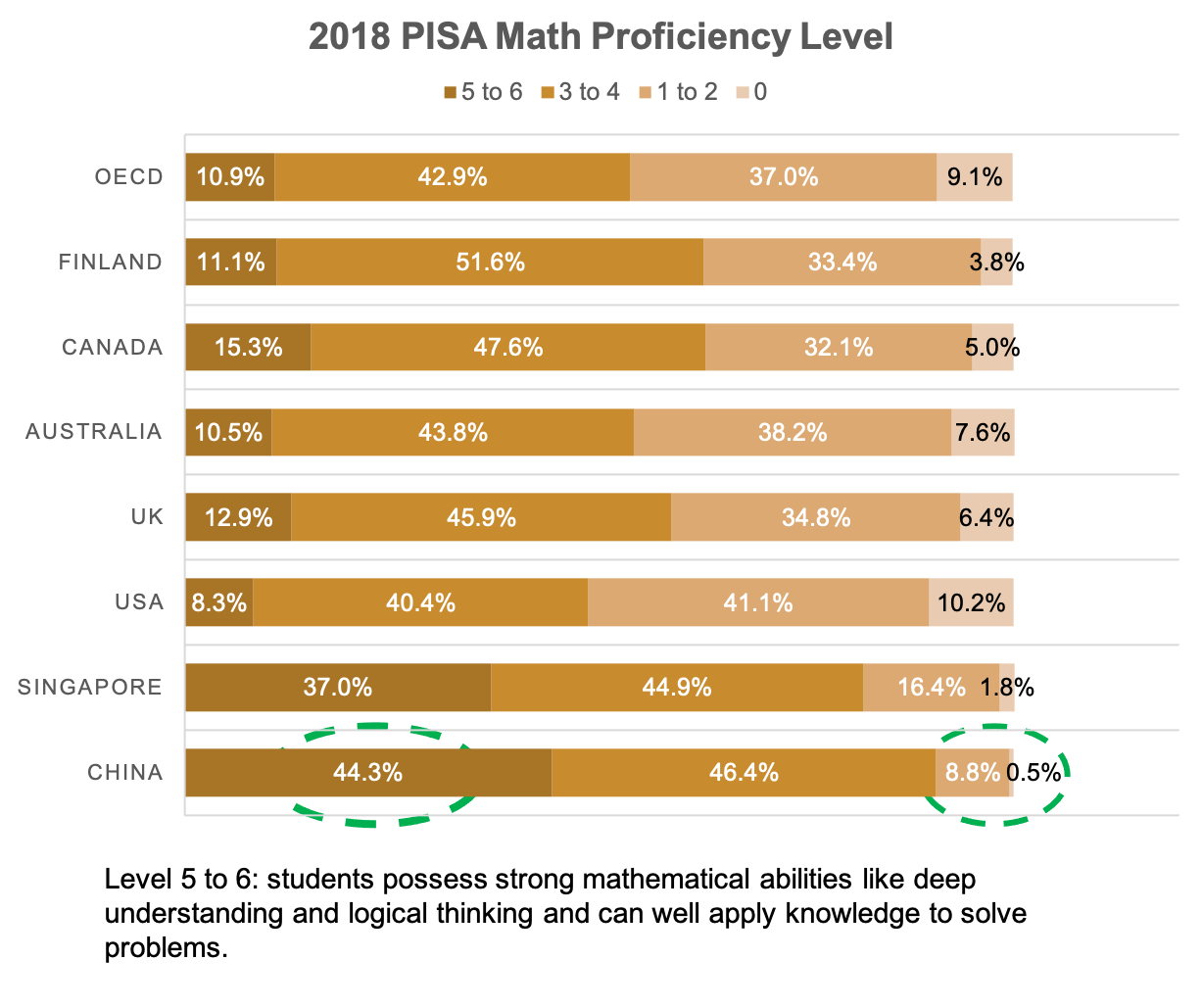
Learning math, just like mastering any life skill, requires a certain amount of practice to achieve expertise. A baby isn’t born with the ability to walk but needs lots of practice to balance the body. To get a driver’s license, a beginner needs months of driving practice to pass the road test, even though he or she may be crystal clear with the rules. Likewise, on top of understanding that solving a quadratic equation is to find the intersection points of the parabola and the x-axis, it still takes intense practice to be adept with all three methods: the quadratic formula, cross multiplication, and completing the squares. In the book Outliers, Gladwell reveals that it takes 10,000 hours to achieve expertise, and it also applies to those widely known geniuses. After Fermat’s Last Theorem was published in 1670, it remained unproved for more than 300 years, until Professor Andrew Wiles finally published his proof in 1995 (Wiles). He is definitely a genius in mathematics, yet he spent decades of consistent effort on proving the theorem. In addition to gaining expertise, practice enhances understanding. Rote memorization and repetition are often blamed; however, nearly half (44.3%) of Chinese students who took the 2018 PISA Mathematics were at level 5 or level 6, the two highest levels in PISA mathematics, compared to the OECD average of 10.9% (105). The two highest levels show that Chinese students possess strong mathematical abilities, such as deep understanding and logical reasoning, rather than just memorizing those subject-related concepts. Whether genius or not, consistent effort is critical to acquiring skills and gaining expertise.

In the competitive test-oriented education system, students tend to resent the mistakes they make in the pursuit of high grades, and only a few students recognize mistakes as opportunities to strengthen their understanding. In fact, trial and error is a fundamental strategy of problem solving in secondary school mathematics. Taking geometric proof as an example, an experienced geometry teacher may also need to take several tries to determine where to add one or more auxiliary lines before arriving at the correct solution. Surely, students need to avoid those careless mistakes. However, most of the mistakes in secondary school or college mathematics are caused by misunderstanding of concepts and lack of logical reasoning abilities. The question “how many two-digit numbers have at least one digit that is a 4?” requires students to think completely. It’s not easy to include all the cases without repetition. Usually, 44 is the number that many students miss and 04 is not a two-digit number which is easily over counted. Nevertheless, through such mistakes, students learn the logic to identify all cases. Sometimes it is hard to come up with an idea to solve a problem, let alone make a mistake. Students get frustrated if that happens. In terms of success, “no idea” surely is unsatisfactory, but it is not bad at all. Research shows that “unsuccessful retrieval attempts enhance subsequence learning (Kornell et al.). Set the test scores aside, and focus only on learning itself. Understanding that true learning is journey with ups and downs.

The problem is, despite all the advice, students still struggle with mathematics. Peer pressure is one of the main reasons. J.Z. was not the top performer but quite well-performed in mathematics, yet he was still sad about himself for not being the top student. Actually, he was better than the top math student in writing, public speaking, and socializing, but he chose to zoom in on his weakness. Comparing one’s weakness with the strengths of others only makes students more distressed. Human beings are born with equal rights but distinct gifts. One of my undergraduate classmates has been a professor in a top research institute, I am just about to start my research career in mathematics education. My classmate could easily prove the well-known primitive tests used in cryptography, but I can’t even understand the Riemann Hypothesis. Aiming at proving Riemann Hypothesis is unrealistic for me, but this does not prevent me from learning number theory. Regardless of my limitations compared to my classmate, I’m glad to see my progress in mathematical proofs, from Diophantine Equation to Pell Equation and more. Students who suffer from peer pressure are inherently eager to improve. Parents and teachers should encourage teenagers to feel satisfied with the progress in their learning process, no matter how slow it is.

If math ever hurts you or you are resentful of not having a math brain, it may be just because you haven’t developed a positive mental attitude towards learning mathematics. Considering learning a journey of self-growth consisting of intense practice and correction from mistakes can prepare you for the challenges. This mental preparation is not only applied to learning mathematics but also applied to learning in any disciplines. More importantly, in the process of learning, students can develop perseverance and resilience that goes beyond just academic achievements.

Works Cited

Dweck, Carol S. Mindset: The New Psychology of Success, Ballantine Books, 2007.

Gladwell, Malcolm. Outliers: The Story of Success. Back Bay Books, Little, Brown and Company, 2019.

Kornell, Nate, et al. “Unsuccessful Retrieval Attempts Enhance Subsequent Learning.” Journal of Experimental Psychology: Learning, Memory, and Cognition, vol. 35, no. 4, 2009, pp. 989–998, https://doi.org/10.1037/a0015729.

PISA 2018 Results (Volume I): What Students Know and Can Do, PISA, OECD Publishing, Paris, 2019, p.105 https://doi.org/10.1787/5f07c754-en.

Wiles, Andrew. “Modular Elliptic Curves and Fermat’s Last Theorem.” The Annals of Mathematics, vol. 141, no. 3, 1995, p. 443, https://doi.org/10.2307/2118559.